(page 1(2))
|
|
(page 1(2)) |
|
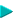
| Introduction | |||||||||||||
|
This web site will discuss the famous Towers of Hanoi puzzle.
We will show its solution, count the number of moves it takes,
introduce recursion, powers of two, induction proofs, and other exciting
and useful mathematical concepts.
Moving Around the Web Site |
The picture in the top left hand corner of the page will take you
to the next page as will the right
arrow | .
The left arrow  will take you
the previous page.
The small Towers of Hanoi picture
in the top and bottom right hand corners will bring you back to
this page.
Legend has it that there is a temple near Hanoi, which is in Vietnam. In this temple there are monks who are solving a giant puzzle consisting of 64 golden rings of different sizes on three diamond needles. To solve the puzzle they must move the entire tower of 64 rings from the starting needle to the ending one. But they can move only one ring at a time, and they may never place a larger ring on top of a smaller one. The legend claims that once the monks are finished, the world will end. So we need to figure out how long it is going to take the monks to finish the puzzle.
Well, actually ... |
this puzzle was invented in 1883 by a
French mathematician called Edouard Lucas.
|
Moving On |
Now you may move to the next page by clicking on the
Towers of Hanoi in the top left hand corner.
When the puzzle pictured there is finished this site will end,
and that's not a legend.
| Please mail any comments or questions to David Tilbrook. Thanks. | ||||||
| hanoi01.qh - 1.6 - 05/10/02 |
|